Page:EB1911 - Volume 05.djvu/281

268

CAPILLARY ACTION

of work, the surface must resist extension, and if the bubble in contracting can do work, the surface must tend to contract. The surface must therefore act like a sheet of india-rubber when extended both in length and breadth, that is, it must exert surface-tension. The tension of the sheet of india-rubber, however, depends on the extent to which it is stretched, and may be different in different directions, whereas the tension of the surface of a liquid remains the same however much the film is extended, and the tension at any point is the same in all directions.

The intensity of this surface-tension is measured by the stress which it exerts across a line of unit length. Let us measure it in the case of the spherical soap-bubble by considering the stress exerted by one hemisphere of the bubble on the other, across the circumference of a great circle. This stress is balanced by the pressure $p$ acting over the area of the same great circle: it is therefore equal to $\pi r^{2}p$. To determine the intensity of the surface-tension we have to divide this quantity by the length of the line across which it acts, which is in this case the circumference of a great circle $2\pi r$. Dividing $\pi r^{2}p$ by this length we obtain ${\tfrac {1}{2}}pr$ as the value of the intensity of the surface-tension, and it is plain from equation 8 that this is equal to ${\mbox{T}}$. Hence the numerical value of the intensity of the surface-tension is equal to the numerical value of the surface-energy per unit of surface. We must remember that since the film has two surfaces the surface-tension of the film is double the tension of the surface of the liquid of which it is formed.

Fig. 9.

To determine the relation between the surface-tension and the pressure which balances it when the form of the surface is not spherical, let us consider the following case:—

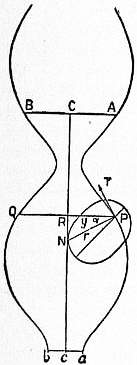
Let fig. 9 represent a section through the axis ${\mbox{C}}c$ of a soap-bubble in the form of a figure of revolution bounded by two circular disks ${\mbox{AB}}$ and $ab$, and having the meridian section ${\mbox{AP}}a$. Let ${\mbox{PQ}}$ Non-spherical soap bubble. be an imaginary section normal to the axis. Let the radius of this section ${\mbox{PR}}$ by $y$, and let ${\mbox{PT}}$, the tangent at ${\mbox{P}}$, make an angle $a$ with the axis.

Let us consider the stresses which are exerted across this imaginary section by the lower part on the upper part. If the internal pressure exceeds the external pressure by $p$, there is in the first place a force $\pi y^{p}$ acting upwards arising from the pressure $p$ over the area of the section. In the next place, there is the surface-tension acting downwards, but at an angle $a$ with the vertical, across the circular section of the bubble itself, whose circumference is $2\pi y$, and the downward force is therefore $2\pi y{\mbox{T}}\cos a$.

Now these forces are balanced by the external force which acts on the disk ${\mbox{ACB}}$, which we may call ${\mbox{F}}$. Hence equating the forces which act on the portion included between ${\mbox{ACB}}$ and ${\mbox{PRQ}}$

$\pi y^{2}p-2\pi y{\mbox{T}}\cos \alpha =-{\mbox{F}}$

(9)

If we make ${\mbox{CR}}=z$, and suppose $z$ to vary, the shape of the bubble of course remaining the same, the values of $y$ and of a will change, but the other quantities will be constant. In studying these variations we may if we please take as our independent variable the length $s$ of the meridian section ${\mbox{AP}}$ reckoned from ${\mbox{A}}$. Differentiating equation 9 with respect to $s$ we obtain, after dividing by $2\pi$ as a common factor,

$py{\frac {dy}{ds}}-{\mbox{T}}\cos \alpha {\frac {dy}{ds}}+{\mbox{T}}y\sin \alpha {\frac {da}{ds}}=0$

(10)

Now

${\frac {dy}{ds}}=\sin \alpha$

(11)

The radius of curvature of the meridian section is

${\mbox{R}}_{1}=-{\frac {ds}{da}}$

(12)

The radius of curvature of a normal section of the surface at right angles to the meridian section is equal to the part of the normal cut off by the axis, which is

${\mbox{R}}_{2}={\mbox{PN}}=y/\cos \alpha$

(13)

Hence dividing equation 10 by $y\sin \alpha$, we find

$p={\mbox{T}}(1/{\mbox{R}}_{1}+1/{\mbox{R}}_{2})$

(14)

This equation, which gives the pressure in terms of the principal radii of curvature, though here proved only in the case of a surface of revolution, must be true of all surfaces. For the curvature of any surface at a given point may be completely defined in terms of the positions of its principal normal sections and their radii of curvature.

Before going further we may deduce from equation 9 the nature of all the figures of revolution which a liquid film can assume. Let us first determine the nature of a curve, such that if it is rolled on the axis its origin will trace out the meridian section of the bubble. Since at any instant the rolling curve is rotating about the point of contact with the axis, the line drawn from this point of contact to the tracing point must be normal to the direction of motion of the tracing point. Hence if N is the point of contact, NP must be normal to the traced curve. Also, since the axis is a tangent to the rolling curve, the ordinate ${\mbox{PR}}$ is the perpendicular from the tracing point ${\mbox{P}}$ on the tangent. Hence the relation between the radius vector and the perpendicular on the tangent of the rolling curve must be identical with the relation between the normal ${\mbox{PN}}$ and the ordinate ${\mbox{PR}}$ of the traced curve. If we write $r$ for ${\mbox{PN}}$, then $y=r\cos \alpha$, and equation 9 becomes

$y^{2}\left(2{\frac {\mbox{T}}{pr}}-1\right)={\frac {\mbox{F}}{\pi p}}.$

This relation between $y$ and $r$ is identical with the relation between the perpendicular from the focus of a conic section on the tangent at a given point and the focal distance of that point, provided the transverse and conjugate axes of the conic are $2a$ and $2b$ respectively, where

$a={\frac {\mbox{T}}{p}},{\mbox{ and }}b^{2}={\frac {\mbox{F}}{\pi p}}.$

Hence the meridian section of the film may be traced by the focus of such a conic, if the conic is made to roll on the axis.

On the different Forms of the Meridian Line.—1. When the conic is an ellipse the meridian line is in the form of a series of waves, and the film itself has a series of alternate swellings and contractions as represented in figs. 9 and 10. This form of the film is called the unduloid.

1a. When the ellipse becomes a circle, the meridian line becomes a straight line parallel to the axis, and the film passes into the form of a cylinder of revolution.

1b. As the ellipse degenerates into the straight line joining its foci, the contracted parts of the unduloid become narrower, till at last the figure becomes a series of spheres in contact.

In all these cases the internal pressure exceeds the external by $2{\mbox{T}}/a$ where $a$ is the semi-transverse axis of the conic. The resultant of the internal pressure and the surface-tension is equivalent to a tension along the axis, and the numerical value of this tension is equal to the force due to the action of this pressure on a circle whose diameter is equal to the conjugate axis of the ellipse.

2. When the conic is a parabola the meridian line is a catenary (fig. 11); the internal pressure is equal to the external pressure, and the tension along the axis is equal to $2\pi {\mbox{T}}m$ where $m$ is the distance of the vertex from the focus.

3. When the conic is a hyperbola the meridian line is in the form of a looped curve (fig. 12). The corresponding figure of the film is called the nodoid. The resultant of the internal pressure and the surface-tension is equivalent to a pressure along the axis equal to that due to a pressure $p$ acting on a circle whose diameter is the conjugate axis of the hyperbola.

When the conjugate axis of the hyperbola is made smaller and smaller, the nodoid approximates more and more to the series of spheres touching each other along the axis. When the conjugate axis of the hyperbola increases without limit, the loops of the nodoid are crowded on one another, and each becomes more nearly a ring of circular section, without, however, ever