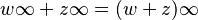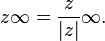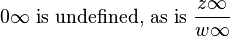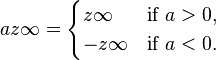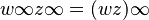Directed infinity

A directed infinity is a type of infinity in the complex plane that has a defined angle θ but an infinite absolute value r. For example, the limit of 1/x where x is a positive number approaching zero is a directed infinity with angle 0; however, 1/0 is not a directed infinity, but a complex infinity. Some rules for manipulation of directed infinities are:

• $w\infty + z\infty = (w+z)\infty$
• $z\infty = \frac{z}{\left | z \right |}\infty.$
• $0\infty\text{ is undefined, as is }\frac{z\infty}{w\infty}$
• $a z\infty = \begin{cases} z\infty & \text{if }a > 0, \\ -z\infty & \text{if }a < 0. \end{cases}$
• $w\infty z\infty = (w z)\infty$

See also

• Point at infinity