Truncated order-4 hexagonal tiling
| Truncated order-4 hexagonal tiling | |
|---|---|
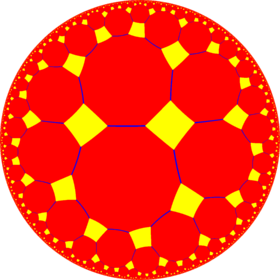 Poincaré disk model of the hyperbolic plane |
|
| Type | Hyperbolic uniform tiling |
| Vertex configuration | 4.12.12 |
| Schläfli symbol | t{6,4} tr{6,6} |
| Wythoff symbol | 2 4 | 6 2 6 6 | |
| Coxeter diagram |     |
| Symmetry group | [6,4], (*642) [6,6], (*662) |
| Dual | Order-6 tetrakis square tiling |
| Properties | Vertex-transitive |
In geometry, the truncated order-4 hexagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t{6,4}. A secondary construction tr{6,6} is called a truncated hexahexagonal tiling with two colors of dodecagons.
Contents
Constructions
There are two uniform constructions of this tiling, first from [6,4] kaleidoscope, and a lower symmetry by removing the last mirror, [6,4,1+], gives [6,6], (*662).
| Name | Tetrahexagonal | Truncated hexahexagonal |
|---|---|---|
| Image |  |
 |
| Symmetry | [6,4] (*642)    |
[6,6] = [6,4,1+] (*662)   =  |
| Symbol | t{6,4} | tr{6,6} |
| Coxeter diagram | |
|
Dual tiling
 |
 |
| The dual tiling, order-6 tetrakis square tiling has face configuration V4.12.12, and represents the fundamental domains of the [6,6] symmetry group. | |
Related polyhedra and tiling
| *n42 symmetry mutation of truncated tilings: 4.2n.2n | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry *n42 [n,4] |
Spherical | Euclidean | Compact hyperbolic | Paracomp. | |||||||
| *242 [2,4] |
*342 [3,4] |
*442 [4,4] |
*542 [5,4] |
*642 [6,4] |
*742 [7,4] |
*842 [8,4]... |
*∞42 [∞,4] |
||||
| Truncated figures |
 |
 |
 |
 |
|
 |
 |
 |
|||
| Config. | 4.4.4 | 4.6.6 | 4.8.8 | 4.10.10 | 4.12.12 | 4.14.14 | 4.16.16 | 4.∞.∞ | |||
| n-kis figures |
 |
 |
 |
 |
|
 |
 |
 |
|||
| Config. | V4.4.4 | V4.6.6 | V4.8.8 | V4.10.10 | V4.12.12 | V4.14.14 | V4.16.16 | V4.∞.∞ | |||
| Uniform tetrahexagonal tilings | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry: [6,4], (*642) (with [6,6] (*662), [(4,3,3)] (*443) , [∞,3,∞] (*3222) index 2 subsymmetries) (And [(∞,3,∞,3)] (*3232) index 4 subsymmetry) |
|||||||||||
=   =   =  |
=  |
= =  = |
= |
=  = =  |
= |
|
|||||
 |
|
 |
 |
 |
 |
 |
|||||
| {6,4} | t{6,4} | r{6,4} | t{4,6} | {4,6} | rr{6,4} | tr{6,4} | |||||
| Uniform duals | |||||||||||
 |
|
|
|
|
|
|
|||||
 |
 |
 |
 |
 |
 |
 |
|||||
| V64 | V4.12.12 | V(4.6)2 | V6.8.8 | V46 | V4.4.4.6 | V4.8.12 | |||||
| Alternations | |||||||||||
| [1+,6,4] (*443) |
[6+,4] (6*2) |
[6,1+,4] (*3222) |
[6,4+] (4*3) |
[6,4,1+] (*662) |
[(6,4,2+)] (2*32) |
[6,4]+ (642) |
|||||
 =  |
 =   |
=   |
= |
=  |
=  |
|
|||||
 |
 |
 |
 |
 |
 |
 |
|||||
| h{6,4} | s{6,4} | hr{6,4} | s{4,6} | h{4,6} | hrr{6,4} | sr{6,4} | |||||
| Uniform hexahexagonal tilings | ||||||
|---|---|---|---|---|---|---|
| Symmetry: [6,6], (*662) | ||||||
=   = |
= = |
= = |
=  = |
= = |
= = |
= = |
 |
 |
 |
 |
 |
 |
 |
| {6,6} = h{4,6} |
t{6,6} = h2{4,6} |
r{6,6} {6,4} |
t{6,6} = h2{4,6} |
{6,6} = h{4,6} |
rr{6,6} r{6,4} |
tr{6,6} t{6,4} |
| Uniform duals | ||||||
|
|
|
|
|
|
|
 |
 |
 |
 |
 |
 |
 |
| V66 | V6.12.12 | V6.6.6.6 | V6.12.12 | V66 | V4.6.4.6 | V4.12.12 |
| Alternations | ||||||
| [1+,6,6] (*663) |
[6+,6] (6*3) |
[6,1+,6] (*3232) |
[6,6+] (6*3) |
[6,6,1+] (*663) |
[(6,6,2+)] (2*33) |
[6,6]+ (662) |
| = |
|
= |
|
=  |
|
|
|
|
|
|
|
|
|
 |
 |
|
|
 |
||
| h{6,6} | s{6,6} | hr{6,6} | s{6,6} | h{6,6} | hrr{6,6} | sr{6,6} |
Symmetry
The dual of the tiling represents the fundamental domains of (*662) orbifold symmetry. From [6,6] (*662) symmetry, there are 15 small index subgroup (12 unique) by mirror removal and alternation operators. Mirrors can be removed if its branch orders are all even, and cuts neighboring branch orders in half. Removing two mirrors leaves a half-order gyration point where the removed mirrors met. In these images fundamental domains are alternately colored black and white, and mirrors exist on the boundaries between colors. The subgroup index-8 group, [1+,6,1+,6,1+] (3333) is the commutator subgroup of [6,6].
Larger subgroup constructed as [6,6*], removing the gyration points of (6*3), index 12 becomes (*333333).
The symmetry can be doubled to 642 symmetry by adding a mirror to bisect the fundamental domain.
| Small index subgroups of [6,6] (*662) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Index | 1 | 2 | 4 | ||||||||
| Diagram | 120px | 120px | 120px | 120px | 120px | 120px | |||||
| Coxeter | [6,6] |
[1+,6,6] =  |
[6,6,1+] = |
[6,1+,6] =   |
[1+,6,6,1+] = |
[6+,6+]  |
|||||
| Orbifold | *662 | *663 | *3232 | *3333 | 33× | ||||||
| Direct subgroups | |||||||||||
| Diagram | 120px | 120px | 120px | 120px | 120px | ||||||
| Coxeter | [6,6+] |
[6+,6] |
[(6,6,2+)] |
[6,1+,6,1+] = = = = |
[1+,6,1+,6] = = =  = |
||||||
| Orbifold | 6*3 | 2*33 | 3*33 | ||||||||
| Direct subgroups | |||||||||||
| Index | 2 | 4 | 8 | ||||||||
| Diagram | 120px | 120px | 120px | 120px | 120px | ||||||
| Coxeter | [6,6]+ |
[6,6+]+ = |
[6+,6]+ = |
[6,1+,6]+ = |
[6+,6+]+ = [1+,6,1+,6]+ = = = |
||||||
| Orbifold | 662 | 663 | 3232 | 3333 | |||||||
| Radical subgroups | |||||||||||
| Index | 12 | 24 | |||||||||
| Diagram | 120px | 120px | 120px | 120px | |||||||
| Coxeter | [6,6*]   |
[6*,6] |
[6,6*]+ |
[6*,6]+ |
|||||||
| Orbifold | *333333 | 333333 | |||||||||
References
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- Lua error in package.lua at line 80: module 'strict' not found.
See also
 |
Wikimedia Commons has media related to Uniform tiling 4-12-12. |
External links
- Weisstein, Eric W., "Hyperbolic tiling", MathWorld.
- Weisstein, Eric W., "Poincaré hyperbolic disk", MathWorld.
- Hyperbolic and Spherical Tiling Gallery
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
- Hyperbolic Planar Tessellations, Don Hatch
|
|
  |
||||||||||||
|
|
|||||||||||||
|
|||||||||||||
|
|||||||||||||
