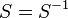Symmetric set

In mathematics, a nonempty subset S of a group G is said to be symmetric if

$S=S^{-1}$

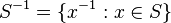where $S^{-1} = \{ x^{-1} : x \in S \}$. In other words, S is symmetric if $x^{-1} \in S$ whenever $x \in S$.

If S is a subset of a vector space, then S is said to be symmetric if it is symmetric with respect to the additive group structure of the vector space; that is, if 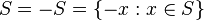$S = -S = \{ -x : x \in S \}$.

Examples

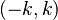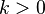• In R, examples of symmetric sets are intervals of the type $(-k, k)$ with $k > 0$, and the sets Z and $\{ -1, 1 \}$.
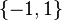• Any vector subspace in a vector space is a symmetric set.
• If S is any subset of a group, then 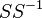$SS^{-1}$ and $S^{-1}S$ are symmetric sets.

References

• R. Cristescu, Topological vector spaces, Noordhoff International Publishing, 1977.
• W. Rudin, Functional Analysis, McGraw-Hill Book Company, 1973.

This article incorporates material from symmetric set on PlanetMath, which is licensed under the Creative Commons Attribution/Share-Alike License.